FreeStatistics.org

Free Statistics

of Irreproducible Research!

Description of Statistical Computation

Author's title

Author*Unverified author*
R Software Modulerwasp_cross.wasp
Title produced by softwareCross Correlation Function
Date of computationThu, 04 Dec 2008 09:44:01 -0700
Cite this page as followsStatistical Computations at FreeStatistics.org, Office for Research Development and Education, URL https://freestatistics.org/blog/index.php?v=date/2008/Dec/04/t1228409070i92gm95uz8ub3kj.htm/, Retrieved Sun, 10 Nov 2024 18:07:37 +0000
Statistical Computations at FreeStatistics.org, Office for Research Development and Education, URL https://freestatistics.org/blog/index.php?pk=28959, Retrieved Sun, 10 Nov 2024 18:07:37 +0000
QR Codes:

Original text written by user:
IsPrivate?No (this computation is public)
User-defined keywords
Estimated Impact208

Tree of Dependent Computations

Family? (F = Feedback message, R = changed R code, M = changed R Module, P = changed Parameters, D = changed Data)
-     [Cross Correlation Function] [] [2008-12-02 21:46:28] [74be16979710d4c4e7c6647856088456]
F   PD  [Cross Correlation Function] [] [2008-12-02 21:49:22] [74be16979710d4c4e7c6647856088456]
-   P       [Cross Correlation Function] [] [2008-12-04 16:44:01] [e02910eed3830f1815f587e12f46cbdb] [Current]
Feedback Forum

Post a new message

Dataset

Dataseries X:
Download CSV
Histogram
Boxplots 
100.8
100.7
86.2
83.2
71.7
77.5
89.8
80.3
78.7
93.8
57.6
60.6
91
85.3
77.4
77.3
68.3
69.9
81.7
75.1
69.9
84
54.3
60
89.9
77
85.3
77.6
69.2
75.5
85.7
72.2
79.9
85.3
52.2
61.2
82.4
85.4
78.2
70.2
70.2
69.3
77.5
66.1
69
79.2
56.2
63.3
77.8
92
78.1
65.1
71.1
70.9
72
81.9
70.6
72.5
65.1
61.1
Dataseries Y:
Download CSV
Histogram 
127.5
128.6
116.6
127.4
105
108.3
125
111.6
106.5
130.3
115
116.1
134
126.5
125.8
136.4
114.9
110.9
125.5
116.8
116.8
125.5
104.2
115.1
132.8
123.3
124.8
122
117.4
117.9
137.4
114.6
124.7
129.6
109.4
120.9
134.9
136.3
133.2
127.2
122.7
120.5
137.8
119.1
124.3
134.4
121.1
122.2
127.7
137.4
132.2
129.2
124.9
124.8
128.2
134.4
118.6
132.6
123.2
112.3

Tables (Output of Computation)





Summary of computational transaction
Raw Inputview raw input (R code)
Raw Outputview raw output of R engine
Computing time0 seconds
R Server'Gwilym Jenkins' @ 72.249.127.135

\begin{tabular}{lllllllll}
\hline
Summary of computational transaction \tabularnewline
Raw Input & view raw input (R code)  \tabularnewline
Raw Output & view raw output of R engine  \tabularnewline
Computing time & 0 seconds \tabularnewline
R Server & 'Gwilym Jenkins' @ 72.249.127.135 \tabularnewline
\hline
\end{tabular}
%Source: https://freestatistics.org/blog/index.php?pk=28959&T=0

[TABLE]
[ROW][C]Summary of computational transaction[/C][/ROW]
[ROW][C]Raw Input[/C][C]view raw input (R code) [/C][/ROW]
[ROW][C]Raw Output[/C][C]view raw output of R engine [/C][/ROW]
[ROW][C]Computing time[/C][C]0 seconds[/C][/ROW]
[ROW][C]R Server[/C][C]'Gwilym Jenkins' @ 72.249.127.135[/C][/ROW]
[/TABLE]
Source: https://freestatistics.org/blog/index.php?pk=28959&T=0

Globally Unique Identifier (entire table): ba.freestatistics.org/blog/index.php?pk=28959&T=0

As an alternative you can also use a QR Code:  
QR Code


The GUIDs for individual cells are displayed in the table below:

Summary of computational transaction
Raw Inputview raw input (R code)
Raw Outputview raw output of R engine
Computing time0 seconds
R Server'Gwilym Jenkins' @ 72.249.127.135






Cross Correlation Function
ParameterValue
Box-Cox transformation parameter (lambda) of X series1
Degree of non-seasonal differencing (d) of X series0
Degree of seasonal differencing (D) of X series1
Seasonal Period (s)12
Box-Cox transformation parameter (lambda) of Y series1
Degree of non-seasonal differencing (d) of Y series0
Degree of seasonal differencing (D) of Y series1
krho(Y[t],X[t+k])
-130.047706289304069
-120.0717327945581489
-110.0860839247701082
-100.0756927888291805
-90.314005641470679
-80.188686419928277
-70.0097338478735242
-60.333861072795509
-50.141099479402920
-4-0.0399525192677145
-30.0614673021249079
-2-0.0263530890909310
-1-0.312726556129722
00.268507528744041
1-0.310261604824478
2-0.241097606665957
30.097374588241768
4-0.266835964361444
5-0.358782164845756
6-0.221989858227328
7-0.183636029749078
8-0.174337917003483
90.0765114353693803
10-0.127834198905980
11-0.0654756097858497
120.213267246906714
130.0121762565168834

\begin{tabular}{lllllllll}
\hline
Cross Correlation Function \tabularnewline
Parameter & Value \tabularnewline
Box-Cox transformation parameter (lambda) of X series & 1 \tabularnewline
Degree of non-seasonal differencing (d) of X series & 0 \tabularnewline
Degree of seasonal differencing (D) of X series & 1 \tabularnewline
Seasonal Period (s) & 12 \tabularnewline
Box-Cox transformation parameter (lambda) of Y series & 1 \tabularnewline
Degree of non-seasonal differencing (d) of Y series & 0 \tabularnewline
Degree of seasonal differencing (D) of Y series & 1 \tabularnewline
k & rho(Y[t],X[t+k]) \tabularnewline
-13 & 0.047706289304069 \tabularnewline
-12 & 0.0717327945581489 \tabularnewline
-11 & 0.0860839247701082 \tabularnewline
-10 & 0.0756927888291805 \tabularnewline
-9 & 0.314005641470679 \tabularnewline
-8 & 0.188686419928277 \tabularnewline
-7 & 0.0097338478735242 \tabularnewline
-6 & 0.333861072795509 \tabularnewline
-5 & 0.141099479402920 \tabularnewline
-4 & -0.0399525192677145 \tabularnewline
-3 & 0.0614673021249079 \tabularnewline
-2 & -0.0263530890909310 \tabularnewline
-1 & -0.312726556129722 \tabularnewline
0 & 0.268507528744041 \tabularnewline
1 & -0.310261604824478 \tabularnewline
2 & -0.241097606665957 \tabularnewline
3 & 0.097374588241768 \tabularnewline
4 & -0.266835964361444 \tabularnewline
5 & -0.358782164845756 \tabularnewline
6 & -0.221989858227328 \tabularnewline
7 & -0.183636029749078 \tabularnewline
8 & -0.174337917003483 \tabularnewline
9 & 0.0765114353693803 \tabularnewline
10 & -0.127834198905980 \tabularnewline
11 & -0.0654756097858497 \tabularnewline
12 & 0.213267246906714 \tabularnewline
13 & 0.0121762565168834 \tabularnewline
\hline
\end{tabular}
%Source: https://freestatistics.org/blog/index.php?pk=28959&T=1

[TABLE]
[ROW][C]Cross Correlation Function[/C][/ROW]
[ROW][C]Parameter[/C][C]Value[/C][/ROW]
[ROW][C]Box-Cox transformation parameter (lambda) of X series[/C][C]1[/C][/ROW]
[ROW][C]Degree of non-seasonal differencing (d) of X series[/C][C]0[/C][/ROW]
[ROW][C]Degree of seasonal differencing (D) of X series[/C][C]1[/C][/ROW]
[ROW][C]Seasonal Period (s)[/C][C]12[/C][/ROW]
[ROW][C]Box-Cox transformation parameter (lambda) of Y series[/C][C]1[/C][/ROW]
[ROW][C]Degree of non-seasonal differencing (d) of Y series[/C][C]0[/C][/ROW]
[ROW][C]Degree of seasonal differencing (D) of Y series[/C][C]1[/C][/ROW]
[ROW][C]k[/C][C]rho(Y[t],X[t+k])[/C][/ROW]
[ROW][C]-13[/C][C]0.047706289304069[/C][/ROW]
[ROW][C]-12[/C][C]0.0717327945581489[/C][/ROW]
[ROW][C]-11[/C][C]0.0860839247701082[/C][/ROW]
[ROW][C]-10[/C][C]0.0756927888291805[/C][/ROW]
[ROW][C]-9[/C][C]0.314005641470679[/C][/ROW]
[ROW][C]-8[/C][C]0.188686419928277[/C][/ROW]
[ROW][C]-7[/C][C]0.0097338478735242[/C][/ROW]
[ROW][C]-6[/C][C]0.333861072795509[/C][/ROW]
[ROW][C]-5[/C][C]0.141099479402920[/C][/ROW]
[ROW][C]-4[/C][C]-0.0399525192677145[/C][/ROW]
[ROW][C]-3[/C][C]0.0614673021249079[/C][/ROW]
[ROW][C]-2[/C][C]-0.0263530890909310[/C][/ROW]
[ROW][C]-1[/C][C]-0.312726556129722[/C][/ROW]
[ROW][C]0[/C][C]0.268507528744041[/C][/ROW]
[ROW][C]1[/C][C]-0.310261604824478[/C][/ROW]
[ROW][C]2[/C][C]-0.241097606665957[/C][/ROW]
[ROW][C]3[/C][C]0.097374588241768[/C][/ROW]
[ROW][C]4[/C][C]-0.266835964361444[/C][/ROW]
[ROW][C]5[/C][C]-0.358782164845756[/C][/ROW]
[ROW][C]6[/C][C]-0.221989858227328[/C][/ROW]
[ROW][C]7[/C][C]-0.183636029749078[/C][/ROW]
[ROW][C]8[/C][C]-0.174337917003483[/C][/ROW]
[ROW][C]9[/C][C]0.0765114353693803[/C][/ROW]
[ROW][C]10[/C][C]-0.127834198905980[/C][/ROW]
[ROW][C]11[/C][C]-0.0654756097858497[/C][/ROW]
[ROW][C]12[/C][C]0.213267246906714[/C][/ROW]
[ROW][C]13[/C][C]0.0121762565168834[/C][/ROW]
[/TABLE]
Source: https://freestatistics.org/blog/index.php?pk=28959&T=1

Globally Unique Identifier (entire table): ba.freestatistics.org/blog/index.php?pk=28959&T=1

As an alternative you can also use a QR Code:  
QR Code


The GUIDs for individual cells are displayed in the table below:

Cross Correlation Function
ParameterValue
Box-Cox transformation parameter (lambda) of X series1
Degree of non-seasonal differencing (d) of X series0
Degree of seasonal differencing (D) of X series1
Seasonal Period (s)12
Box-Cox transformation parameter (lambda) of Y series1
Degree of non-seasonal differencing (d) of Y series0
Degree of seasonal differencing (D) of Y series1
krho(Y[t],X[t+k])
-130.047706289304069
-120.0717327945581489
-110.0860839247701082
-100.0756927888291805
-90.314005641470679
-80.188686419928277
-70.0097338478735242
-60.333861072795509
-50.141099479402920
-4-0.0399525192677145
-30.0614673021249079
-2-0.0263530890909310
-1-0.312726556129722
00.268507528744041
1-0.310261604824478
2-0.241097606665957
30.097374588241768
4-0.266835964361444
5-0.358782164845756
6-0.221989858227328
7-0.183636029749078
8-0.174337917003483
90.0765114353693803
10-0.127834198905980
11-0.0654756097858497
120.213267246906714
130.0121762565168834


Figures (Output of Computation)

Input Parameters & R Code

Parameters (Session):
par1 = 36 ; par2 = 1 ; par3 = 1 ; par4 = 1 ; par5 = 12 ;
Parameters (R input):
par1 = 1 ; par2 = 0 ; par3 = 1 ; par4 = 12 ; par5 = 1 ; par6 = 0 ; par7 = 1 ;
R code (references can be found in the software module):
par1 <- as.numeric(par1)
par2 <- as.numeric(par2)
par3 <- as.numeric(par3)
par4 <- as.numeric(par4)
par5 <- as.numeric(par5)
par6 <- as.numeric(par6)
par7 <- as.numeric(par7)
if (par1 == 0) {
x <- log(x)
} else {
x <- (x ^ par1 - 1) / par1
}
if (par5 == 0) {
y <- log(y)
} else {
y <- (y ^ par5 - 1) / par5
}
if (par2 > 0) x <- diff(x,lag=1,difference=par2)
if (par6 > 0) y <- diff(y,lag=1,difference=par6)
if (par3 > 0) x <- diff(x,lag=par4,difference=par3)
if (par7 > 0) y <- diff(y,lag=par4,difference=par7)
x
y
bitmap(file='test1.png')
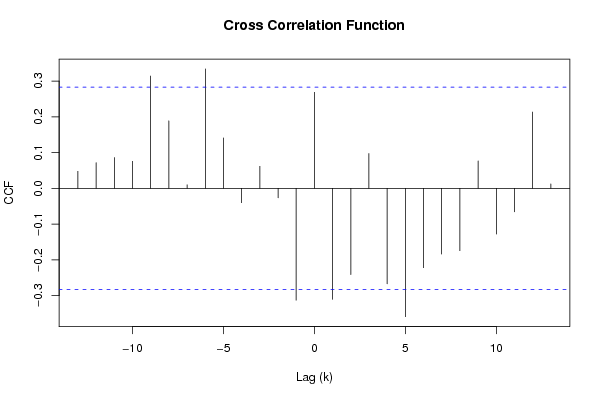
(r <- ccf(x,y,main='Cross Correlation Function',ylab='CCF',xlab='Lag (k)'))
dev.off()
load(file='createtable')
a<-table.start()
a<-table.row.start(a)
a<-table.element(a,'Cross Correlation Function',2,TRUE)
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'Parameter',header=TRUE)
a<-table.element(a,'Value',header=TRUE)
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'Box-Cox transformation parameter (lambda) of X series',header=TRUE)
a<-table.element(a,par1)
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'Degree of non-seasonal differencing (d) of X series',header=TRUE)
a<-table.element(a,par2)
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'Degree of seasonal differencing (D) of X series',header=TRUE)
a<-table.element(a,par3)
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'Seasonal Period (s)',header=TRUE)
a<-table.element(a,par4)
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'Box-Cox transformation parameter (lambda) of Y series',header=TRUE)
a<-table.element(a,par5)
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'Degree of non-seasonal differencing (d) of Y series',header=TRUE)
a<-table.element(a,par6)
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'Degree of seasonal differencing (D) of Y series',header=TRUE)
a<-table.element(a,par7)
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'k',header=TRUE)
a<-table.element(a,'rho(Y[t],X[t+k])',header=TRUE)
a<-table.row.end(a)
mylength <- length(r$acf)
myhalf <- floor((mylength-1)/2)
for (i in 1:mylength) {
a<-table.row.start(a)
a<-table.element(a,i-myhalf-1,header=TRUE)
a<-table.element(a,r$acf[i])
a<-table.row.end(a)
}
a<-table.end(a)
table.save(a,file='mytable.tab')