FreeStatistics.org

Free Statistics

of Irreproducible Research!

Description of Statistical Computation

Author's title

Author*The author of this computation has been verified*
R Software Modulerwasp_smp.wasp
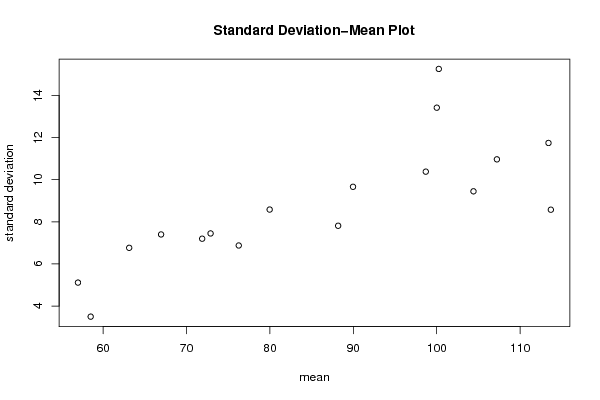
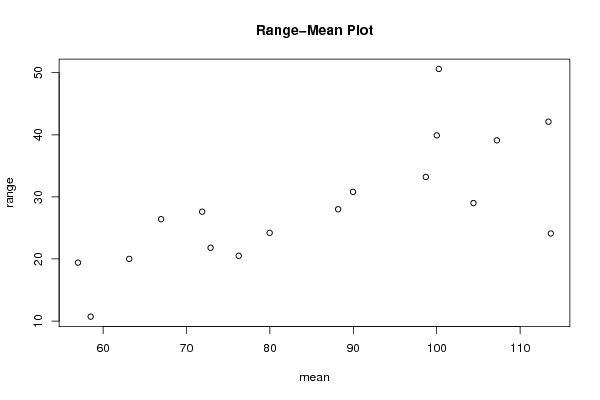
Title produced by softwareStandard Deviation-Mean Plot
Date of computationFri, 22 Dec 2017 11:46:11 +0100
Cite this page as followsStatistical Computations at FreeStatistics.org, Office for Research Development and Education, URL https://freestatistics.org/blog/index.php?v=date/2017/Dec/22/t1513940986ckkei4iawydd3ee.htm/, Retrieved Thu, 31 Oct 2024 22:48:17 +0000
Statistical Computations at FreeStatistics.org, Office for Research Development and Education, URL https://freestatistics.org/blog/index.php?pk=310761, Retrieved Thu, 31 Oct 2024 22:48:17 +0000
QR Codes:

Original text written by user:
IsPrivate?No (this computation is public)
User-defined keywords
Estimated Impact120

Tree of Dependent Computations

Family? (F = Feedback message, R = changed R code, M = changed R Module, P = changed Parameters, D = changed Data)
-       [Standard Deviation-Mean Plot] [] [2017-12-22 10:46:11] [30e08ccf92bdf95f8dcbf6f321363364] [Current]
Feedback Forum

Post a new message

Dataset

Dataseries X:
Download CSV
Histogram
Boxplots 
46,8
52,8
58,3
54,5
64,7
58,3
57,5
56,7
56
66,2
58,2
53,9
53,1
54,4
59,2
57,8
61,5
60,1
60,1
58,4
56,8
63,8
53,9
63,1
55,7
54,9
64,6
60,2
63,9
69,9
58,5
52
66,7
72
68,4
70,8
56,5
62,6
66,5
69,2
63,7
73,6
64,1
53,8
72,2
80,2
69,1
72
66,3
72,5
88,9
88,6
73,7
86
70
71,6
90,5
85,7
84,8
81,1
70,8
65,7
86,2
76,1
79,8
85,2
75,8
69,4
85
75
77,7
68,5
68,4
65
73,2
67,9
76,5
85,5
71,7
57,9
75,5
78,2
75,7
67,1
74,6
66,2
74,9
69,5
76,1
82,3
82,1
60,5
71,2
81,4
74,5
61,4
83,8
85,4
91,6
91,9
86,3
96,8
81
70,8
98,8
94,5
84,5
92,8
81,2
75,7
86,7
87,5
87,8
103,1
96,4
77,1
106,5
95,7
95,3
86,6
89,6
81,9
98,4
92,9
83,9
121,8
103,9
87,5
118,9
109
112,2
100,1
111,3
102,7
122,6
124,8
120,3
118,3
108,7
100,7
124
103,1
115
112,7
101,7
111,5
114,4
112,5
107,2
136,7
107,8
94,6
110,7
126,6
127,9
109,2
87,1
90,8
94,5
103,3
103,2
105,4
103,9
79,8
105,6
113
87,7
110
90,3
108,9
105,1
113
100,4
110,1
114,7
88,6
117,2
127,7
107,8
102,8
100,2
108,4
114,2
94,4
92,2
115,3
102
86,3
112
112,5
109,5
105,9
115,3
126,2
112,2
112,5
106,9
90,6
75,6
78,8
101,8
93,9
100
89,2
97,7
121,1
108,8
92,9
113,6
112,6
98,8
78

Tables (Output of Computation)





Summary of computational transaction
Raw Input view raw input (R code)
Raw Outputview raw output of R engine
Computing time1 seconds
R ServerBig Analytics Cloud Computing Center

\begin{tabular}{lllllllll}
\hline
Summary of computational transaction \tabularnewline
Raw Input view raw input (R code)  \tabularnewline
Raw Outputview raw output of R engine  \tabularnewline
Computing time1 seconds \tabularnewline
R ServerBig Analytics Cloud Computing Center \tabularnewline
\hline
\end{tabular}
%Source: https://freestatistics.org/blog/index.php?pk=310761&T=0

[TABLE]
[ROW]
Summary of computational transaction[/C][/ROW] [ROW]Raw Input[/C] view raw input (R code) [/C][/ROW] [ROW]Raw Output[/C]view raw output of R engine [/C][/ROW] [ROW]Computing time[/C]1 seconds[/C][/ROW] [ROW]R Server[/C]Big Analytics Cloud Computing Center[/C][/ROW] [/TABLE] Source: https://freestatistics.org/blog/index.php?pk=310761&T=0

Globally Unique Identifier (entire table): ba.freestatistics.org/blog/index.php?pk=310761&T=0

As an alternative you can also use a QR Code:  
QR Code


The GUIDs for individual cells are displayed in the table below:

Summary of computational transaction
Raw Input view raw input (R code)
Raw Outputview raw output of R engine
Computing time1 seconds
R ServerBig Analytics Cloud Computing Center






Standard Deviation-Mean Plot
SectionMeanStandard DeviationRange
156.99166666666675.1135218842404619.4
258.51666666666673.4951481522334110.7
363.13333333333336.7651961381676720
466.95833333333337.4006705265417826.4
579.9758.5829773388958724.2
676.26666666666676.8764860652851320.5
771.88333333333337.1974532532926827.6
872.89166666666677.4485457193557821.8
988.18333333333337.8132560240254328
1089.96666666666679.6637404139328430.8
11100.00833333333313.42219724143139.9
12113.6833333333338.5760589945958224.1
13113.411.746798403278842.1
1498.691666666666710.383330901308133.2
15107.21666666666710.968453386734639.1
16104.4083333333339.4500080166712829
17100.2515.263533488201750.6

\begin{tabular}{lllllllll}
\hline
Standard Deviation-Mean Plot \tabularnewline
Section & Mean & Standard Deviation & Range \tabularnewline
1 & 56.9916666666667 & 5.11352188424046 & 19.4 \tabularnewline
2 & 58.5166666666667 & 3.49514815223341 & 10.7 \tabularnewline
3 & 63.1333333333333 & 6.76519613816767 & 20 \tabularnewline
4 & 66.9583333333333 & 7.40067052654178 & 26.4 \tabularnewline
5 & 79.975 & 8.58297733889587 & 24.2 \tabularnewline
6 & 76.2666666666667 & 6.87648606528513 & 20.5 \tabularnewline
7 & 71.8833333333333 & 7.19745325329268 & 27.6 \tabularnewline
8 & 72.8916666666667 & 7.44854571935578 & 21.8 \tabularnewline
9 & 88.1833333333333 & 7.81325602402543 & 28 \tabularnewline
10 & 89.9666666666667 & 9.66374041393284 & 30.8 \tabularnewline
11 & 100.008333333333 & 13.422197241431 & 39.9 \tabularnewline
12 & 113.683333333333 & 8.57605899459582 & 24.1 \tabularnewline
13 & 113.4 & 11.7467984032788 & 42.1 \tabularnewline
14 & 98.6916666666667 & 10.3833309013081 & 33.2 \tabularnewline
15 & 107.216666666667 & 10.9684533867346 & 39.1 \tabularnewline
16 & 104.408333333333 & 9.45000801667128 & 29 \tabularnewline
17 & 100.25 & 15.2635334882017 & 50.6 \tabularnewline
\hline
\end{tabular}
%Source: https://freestatistics.org/blog/index.php?pk=310761&T=1

[TABLE]
[ROW][C]Standard Deviation-Mean Plot[/C][/ROW]
[ROW][C]Section[/C][C]Mean[/C][C]Standard Deviation[/C][C]Range[/C][/ROW]
[ROW][C]1[/C][C]56.9916666666667[/C][C]5.11352188424046[/C][C]19.4[/C][/ROW]
[ROW][C]2[/C][C]58.5166666666667[/C][C]3.49514815223341[/C][C]10.7[/C][/ROW]
[ROW][C]3[/C][C]63.1333333333333[/C][C]6.76519613816767[/C][C]20[/C][/ROW]
[ROW][C]4[/C][C]66.9583333333333[/C][C]7.40067052654178[/C][C]26.4[/C][/ROW]
[ROW][C]5[/C][C]79.975[/C][C]8.58297733889587[/C][C]24.2[/C][/ROW]
[ROW][C]6[/C][C]76.2666666666667[/C][C]6.87648606528513[/C][C]20.5[/C][/ROW]
[ROW][C]7[/C][C]71.8833333333333[/C][C]7.19745325329268[/C][C]27.6[/C][/ROW]
[ROW][C]8[/C][C]72.8916666666667[/C][C]7.44854571935578[/C][C]21.8[/C][/ROW]
[ROW][C]9[/C][C]88.1833333333333[/C][C]7.81325602402543[/C][C]28[/C][/ROW]
[ROW][C]10[/C][C]89.9666666666667[/C][C]9.66374041393284[/C][C]30.8[/C][/ROW]
[ROW][C]11[/C][C]100.008333333333[/C][C]13.422197241431[/C][C]39.9[/C][/ROW]
[ROW][C]12[/C][C]113.683333333333[/C][C]8.57605899459582[/C][C]24.1[/C][/ROW]
[ROW][C]13[/C][C]113.4[/C][C]11.7467984032788[/C][C]42.1[/C][/ROW]
[ROW][C]14[/C][C]98.6916666666667[/C][C]10.3833309013081[/C][C]33.2[/C][/ROW]
[ROW][C]15[/C][C]107.216666666667[/C][C]10.9684533867346[/C][C]39.1[/C][/ROW]
[ROW][C]16[/C][C]104.408333333333[/C][C]9.45000801667128[/C][C]29[/C][/ROW]
[ROW][C]17[/C][C]100.25[/C][C]15.2635334882017[/C][C]50.6[/C][/ROW]
[/TABLE]
Source: https://freestatistics.org/blog/index.php?pk=310761&T=1

Globally Unique Identifier (entire table): ba.freestatistics.org/blog/index.php?pk=310761&T=1

As an alternative you can also use a QR Code:  
QR Code


The GUIDs for individual cells are displayed in the table below:

Standard Deviation-Mean Plot
SectionMeanStandard DeviationRange
156.99166666666675.1135218842404619.4
258.51666666666673.4951481522334110.7
363.13333333333336.7651961381676720
466.95833333333337.4006705265417826.4
579.9758.5829773388958724.2
676.26666666666676.8764860652851320.5
771.88333333333337.1974532532926827.6
872.89166666666677.4485457193557821.8
988.18333333333337.8132560240254328
1089.96666666666679.6637404139328430.8
11100.00833333333313.42219724143139.9
12113.6833333333338.5760589945958224.1
13113.411.746798403278842.1
1498.691666666666710.383330901308133.2
15107.21666666666710.968453386734639.1
16104.4083333333339.4500080166712829
17100.2515.263533488201750.6






Regression: S.E.(k) = alpha + beta * Mean(k)
alpha-1.37866111080563
beta0.118710097838787
S.D.0.0248620699026243
T-STAT4.77474716722024
p-value0.000245770615032284

\begin{tabular}{lllllllll}
\hline
Regression: S.E.(k) = alpha + beta * Mean(k) \tabularnewline
alpha & -1.37866111080563 \tabularnewline
beta & 0.118710097838787 \tabularnewline
S.D. & 0.0248620699026243 \tabularnewline
T-STAT & 4.77474716722024 \tabularnewline
p-value & 0.000245770615032284 \tabularnewline
\hline
\end{tabular}
%Source: https://freestatistics.org/blog/index.php?pk=310761&T=2

[TABLE]
[ROW][C]Regression: S.E.(k) = alpha + beta * Mean(k)[/C][/ROW]
[ROW][C]alpha[/C][C]-1.37866111080563[/C][/ROW]
[ROW][C]beta[/C][C]0.118710097838787[/C][/ROW]
[ROW][C]S.D.[/C][C]0.0248620699026243[/C][/ROW]
[ROW][C]T-STAT[/C][C]4.77474716722024[/C][/ROW]
[ROW][C]p-value[/C][C]0.000245770615032284[/C][/ROW]
[/TABLE]
Source: https://freestatistics.org/blog/index.php?pk=310761&T=2

Globally Unique Identifier (entire table): ba.freestatistics.org/blog/index.php?pk=310761&T=2

As an alternative you can also use a QR Code:  
QR Code


The GUIDs for individual cells are displayed in the table below:

Regression: S.E.(k) = alpha + beta * Mean(k)
alpha-1.37866111080563
beta0.118710097838787
S.D.0.0248620699026243
T-STAT4.77474716722024
p-value0.000245770615032284






Regression: ln S.E.(k) = alpha + beta * ln Mean(k)
alpha-3.5068530549408
beta1.27087506357934
S.D.0.220452288496407
T-STAT5.76485312194914
p-value3.73414704513073e-05
Lambda-0.270875063579344

\begin{tabular}{lllllllll}
\hline
Regression: ln S.E.(k) = alpha + beta * ln Mean(k) \tabularnewline
alpha & -3.5068530549408 \tabularnewline
beta & 1.27087506357934 \tabularnewline
S.D. & 0.220452288496407 \tabularnewline
T-STAT & 5.76485312194914 \tabularnewline
p-value & 3.73414704513073e-05 \tabularnewline
Lambda & -0.270875063579344 \tabularnewline
\hline
\end{tabular}
%Source: https://freestatistics.org/blog/index.php?pk=310761&T=3

[TABLE]
[ROW][C]Regression: ln S.E.(k) = alpha + beta * ln Mean(k)[/C][/ROW]
[ROW][C]alpha[/C][C]-3.5068530549408[/C][/ROW]
[ROW][C]beta[/C][C]1.27087506357934[/C][/ROW]
[ROW][C]S.D.[/C][C]0.220452288496407[/C][/ROW]
[ROW][C]T-STAT[/C][C]5.76485312194914[/C][/ROW]
[ROW][C]p-value[/C][C]3.73414704513073e-05[/C][/ROW]
[ROW][C]Lambda[/C][C]-0.270875063579344[/C][/ROW]
[/TABLE]
Source: https://freestatistics.org/blog/index.php?pk=310761&T=3

Globally Unique Identifier (entire table): ba.freestatistics.org/blog/index.php?pk=310761&T=3

As an alternative you can also use a QR Code:  
QR Code


The GUIDs for individual cells are displayed in the table below:

Regression: ln S.E.(k) = alpha + beta * ln Mean(k)
alpha-3.5068530549408
beta1.27087506357934
S.D.0.220452288496407
T-STAT5.76485312194914
p-value3.73414704513073e-05
Lambda-0.270875063579344


Figures (Output of Computation)

Input Parameters & R Code

Parameters (Session):
Parameters (R input):
par1 = 12 ;
R code (references can be found in the software module):
par1 <- as.numeric(par1)
(n <- length(x))
(np <- floor(n / par1))
arr <- array(NA,dim=c(par1,np))
j <- 0
k <- 1
for (i in 1:(np*par1))
{
j = j + 1
arr[j,k] <- x[i]
if (j == par1) {
j = 0
k=k+1
}
}
arr
arr.mean <- array(NA,dim=np)
arr.sd <- array(NA,dim=np)
arr.range <- array(NA,dim=np)
for (j in 1:np)
{
arr.mean[j] <- mean(arr[,j],na.rm=TRUE)
arr.sd[j] <- sd(arr[,j],na.rm=TRUE)
arr.range[j] <- max(arr[,j],na.rm=TRUE) - min(arr[,j],na.rm=TRUE)
}
arr.mean
arr.sd
arr.range
(lm1 <- lm(arr.sd~arr.mean))
(lnlm1 <- lm(log(arr.sd)~log(arr.mean)))
(lm2 <- lm(arr.range~arr.mean))
bitmap(file='test1.png')
plot(arr.mean,arr.sd,main='Standard Deviation-Mean Plot',xlab='mean',ylab='standard deviation')
dev.off()
bitmap(file='test2.png')
plot(arr.mean,arr.range,main='Range-Mean Plot',xlab='mean',ylab='range')
dev.off()
load(file='createtable')
a<-table.start()
a<-table.row.start(a)
a<-table.element(a,'Standard Deviation-Mean Plot',4,TRUE)
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'Section',header=TRUE)
a<-table.element(a,'Mean',header=TRUE)
a<-table.element(a,'Standard Deviation',header=TRUE)
a<-table.element(a,'Range',header=TRUE)
a<-table.row.end(a)
for (j in 1:np) {
a<-table.row.start(a)
a<-table.element(a,j,header=TRUE)
a<-table.element(a,arr.mean[j])
a<-table.element(a,arr.sd[j] )
a<-table.element(a,arr.range[j] )
a<-table.row.end(a)
}
a<-table.end(a)
table.save(a,file='mytable.tab')
a<-table.start()
a<-table.row.start(a)
a<-table.element(a,'Regression: S.E.(k) = alpha + beta * Mean(k)',2,TRUE)
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'alpha',header=TRUE)
a<-table.element(a,lm1$coefficients[[1]])
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'beta',header=TRUE)
a<-table.element(a,lm1$coefficients[[2]])
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'S.D.',header=TRUE)
a<-table.element(a,summary(lm1)$coefficients[2,2])
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'T-STAT',header=TRUE)
a<-table.element(a,summary(lm1)$coefficients[2,3])
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'p-value',header=TRUE)
a<-table.element(a,summary(lm1)$coefficients[2,4])
a<-table.row.end(a)
a<-table.end(a)
table.save(a,file='mytable1.tab')
a<-table.start()
a<-table.row.start(a)
a<-table.element(a,'Regression: ln S.E.(k) = alpha + beta * ln Mean(k)',2,TRUE)
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'alpha',header=TRUE)
a<-table.element(a,lnlm1$coefficients[[1]])
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'beta',header=TRUE)
a<-table.element(a,lnlm1$coefficients[[2]])
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'S.D.',header=TRUE)
a<-table.element(a,summary(lnlm1)$coefficients[2,2])
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'T-STAT',header=TRUE)
a<-table.element(a,summary(lnlm1)$coefficients[2,3])
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'p-value',header=TRUE)
a<-table.element(a,summary(lnlm1)$coefficients[2,4])
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'Lambda',header=TRUE)
a<-table.element(a,1-lnlm1$coefficients[[2]])
a<-table.row.end(a)
a<-table.end(a)
table.save(a,file='mytable2.tab')