Repository of Reproducible Computations

Free Statistics

of Irreproducible Research!

Author's title

Author

*The author of this computation has been verified*

R Software Module

rwasp_smp.wasp

Title produced by software

Standard Deviation-Mean Plot

Date of computation

Fri, 05 Dec 2008 07:59:18 -0700

Cite this page as follows

Statistical Computations at FreeStatistics.org, Office for Research Development and Education, URL https://freestatistics.org/blog/index.php?v=date/2008/Dec/05/t12284985214ao7ppqnlveexta.htm/, Retrieved Sun, 10 Nov 2024 17:56:07 +0000

Statistical Computations at FreeStatistics.org, Office for Research Development and Education, URL https://freestatistics.org/blog/index.php?pk=29358, Retrieved Sun, 10 Nov 2024 17:56:07 +0000

QR Codes:

Paste this QR Code to cite your computation.

Original text written by user:

IsPrivate?

No (this computation is public)

User-defined keywords

Estimated Impact

204

Family? (F = Feedback message, R = changed R code, M = changed R Module, P = changed Parameters, D = changed Data)

-       [Standard Deviation-Mean Plot] [inv] [2008-12-05 14:59:18] [fa8b44cd657c07c6ee11bb2476ca3f8d] [Current]
-   P     [Standard Deviation-Mean Plot] [SDMP inv] [2008-12-21 14:54:19] [fad8a251ac01c156a8ae23a83577546f] 
-   PD    [Standard Deviation-Mean Plot] [SDMP duur cons] [2008-12-21 15:01:25] [fad8a251ac01c156a8ae23a83577546f] 
-   PD    [Standard Deviation-Mean Plot] [SDMP nt duur cons] [2008-12-21 15:08:14] [fad8a251ac01c156a8ae23a83577546f] 
-   PD    [Standard Deviation-Mean Plot] [SDMP cons] [2008-12-21 15:14:27] [fad8a251ac01c156a8ae23a83577546f] 

Feedback Forum

Post a new message

Dataseries X:

Download CSV

Histogram

Boxplots

Summary of computational transaction
Raw Input	view raw input (R code)
Raw Output	view raw output of R engine
Computing time	1 seconds
R Server	'Gwilym Jenkins' @ 72.249.127.135

\begin{tabular}{lllllllll}
\hline
Summary of computational transaction \tabularnewline
Raw Input & view raw input (R code)  \tabularnewline
Raw Output & view raw output of R engine  \tabularnewline
Computing time & 1 seconds \tabularnewline
R Server & 'Gwilym Jenkins' @ 72.249.127.135 \tabularnewline
\hline
\end{tabular}
%Source: https://freestatistics.org/blog/index.php?pk=29358&T=0

[TABLE]
[ROW][C]Summary of computational transaction[/C][/ROW]
[ROW][C]Raw Input[/C][C]view raw input (R code) [/C][/ROW]
[ROW][C]Raw Output[/C][C]view raw output of R engine [/C][/ROW]
[ROW][C]Computing time[/C][C]1 seconds[/C][/ROW]
[ROW][C]R Server[/C][C]'Gwilym Jenkins' @ 72.249.127.135[/C][/ROW]
[/TABLE]
Source: https://freestatistics.org/blog/index.php?pk=29358&T=0

Globally Unique Identifier (entire table): ba.freestatistics.org/blog/index.php?pk=29358&T=0

As an alternative you can also use a QR Code:

The GUIDs for individual cells are displayed in the table below:

Summary of computational transaction
Raw Input	view raw input (R code)
Raw Output	view raw output of R engine
Computing time	1 seconds
R Server	'Gwilym Jenkins' @ 72.249.127.135

Standard Deviation-Mean Plot
Section	Mean	Standard Deviation	Range
1	104.125	9.6060310916285	19.2
2	93.675	15.8125214097352	35.4
3	112.675	3.49892840738418	7.4
4	110.475	16.6520018816557	37.3
5	100.625	21.2528233418527	46.2
6	116.5	5.99722157892025	12.1
7	113.25	12.8743931895837	28.5
8	104.375	18.7010472077546	41.8
9	117.725	4.89378858009484	11.9
10	117.125	17.0308298877849	37.7
11	112.125	22.095154069011	44.8
12	128.35	6.54038734836605	13.7000000000000
13	116.05	7.08119575589698	15.2
14	115.05	20.5929599620841	45
15	129.925	5.34314202194427	13

\begin{tabular}{lllllllll}
\hline
Standard Deviation-Mean Plot \tabularnewline
Section & Mean & Standard Deviation & Range \tabularnewline
1 & 104.125 & 9.6060310916285 & 19.2 \tabularnewline
2 & 93.675 & 15.8125214097352 & 35.4 \tabularnewline
3 & 112.675 & 3.49892840738418 & 7.4 \tabularnewline
4 & 110.475 & 16.6520018816557 & 37.3 \tabularnewline
5 & 100.625 & 21.2528233418527 & 46.2 \tabularnewline
6 & 116.5 & 5.99722157892025 & 12.1 \tabularnewline
7 & 113.25 & 12.8743931895837 & 28.5 \tabularnewline
8 & 104.375 & 18.7010472077546 & 41.8 \tabularnewline
9 & 117.725 & 4.89378858009484 & 11.9 \tabularnewline
10 & 117.125 & 17.0308298877849 & 37.7 \tabularnewline
11 & 112.125 & 22.095154069011 & 44.8 \tabularnewline
12 & 128.35 & 6.54038734836605 & 13.7000000000000 \tabularnewline
13 & 116.05 & 7.08119575589698 & 15.2 \tabularnewline
14 & 115.05 & 20.5929599620841 & 45 \tabularnewline
15 & 129.925 & 5.34314202194427 & 13 \tabularnewline
\hline
\end{tabular}
%Source: https://freestatistics.org/blog/index.php?pk=29358&T=1

[TABLE]
[ROW][C]Standard Deviation-Mean Plot[/C][/ROW]
[ROW][C]Section[/C][C]Mean[/C][C]Standard Deviation[/C][C]Range[/C][/ROW]
[ROW][C]1[/C][C]104.125[/C][C]9.6060310916285[/C][C]19.2[/C][/ROW]
[ROW][C]2[/C][C]93.675[/C][C]15.8125214097352[/C][C]35.4[/C][/ROW]
[ROW][C]3[/C][C]112.675[/C][C]3.49892840738418[/C][C]7.4[/C][/ROW]
[ROW][C]4[/C][C]110.475[/C][C]16.6520018816557[/C][C]37.3[/C][/ROW]
[ROW][C]5[/C][C]100.625[/C][C]21.2528233418527[/C][C]46.2[/C][/ROW]
[ROW][C]6[/C][C]116.5[/C][C]5.99722157892025[/C][C]12.1[/C][/ROW]
[ROW][C]7[/C][C]113.25[/C][C]12.8743931895837[/C][C]28.5[/C][/ROW]
[ROW][C]8[/C][C]104.375[/C][C]18.7010472077546[/C][C]41.8[/C][/ROW]
[ROW][C]9[/C][C]117.725[/C][C]4.89378858009484[/C][C]11.9[/C][/ROW]
[ROW][C]10[/C][C]117.125[/C][C]17.0308298877849[/C][C]37.7[/C][/ROW]
[ROW][C]11[/C][C]112.125[/C][C]22.095154069011[/C][C]44.8[/C][/ROW]
[ROW][C]12[/C][C]128.35[/C][C]6.54038734836605[/C][C]13.7000000000000[/C][/ROW]
[ROW][C]13[/C][C]116.05[/C][C]7.08119575589698[/C][C]15.2[/C][/ROW]
[ROW][C]14[/C][C]115.05[/C][C]20.5929599620841[/C][C]45[/C][/ROW]
[ROW][C]15[/C][C]129.925[/C][C]5.34314202194427[/C][C]13[/C][/ROW]
[/TABLE]
Source: https://freestatistics.org/blog/index.php?pk=29358&T=1

Globally Unique Identifier (entire table): ba.freestatistics.org/blog/index.php?pk=29358&T=1

As an alternative you can also use a QR Code:

The GUIDs for individual cells are displayed in the table below:

Standard Deviation-Mean Plot
Section	Mean	Standard Deviation	Range
1	104.125	9.6060310916285	19.2
2	93.675	15.8125214097352	35.4
3	112.675	3.49892840738418	7.4
4	110.475	16.6520018816557	37.3
5	100.625	21.2528233418527	46.2
6	116.5	5.99722157892025	12.1
7	113.25	12.8743931895837	28.5
8	104.375	18.7010472077546	41.8
9	117.725	4.89378858009484	11.9
10	117.125	17.0308298877849	37.7
11	112.125	22.095154069011	44.8
12	128.35	6.54038734836605	13.7000000000000
13	116.05	7.08119575589698	15.2
14	115.05	20.5929599620841	45
15	129.925	5.34314202194427	13

Regression: S.E.(k) = alpha + beta * Mean(k)
alpha	54.0208961852103
beta	-0.367802970978669
S.D.	0.165739886865321
T-STAT	-2.21915784989973
p-value	0.0448883210996267

\begin{tabular}{lllllllll}
\hline
Regression: S.E.(k) = alpha + beta * Mean(k) \tabularnewline
alpha & 54.0208961852103 \tabularnewline
beta & -0.367802970978669 \tabularnewline
S.D. & 0.165739886865321 \tabularnewline
T-STAT & -2.21915784989973 \tabularnewline
p-value & 0.0448883210996267 \tabularnewline
\hline
\end{tabular}
%Source: https://freestatistics.org/blog/index.php?pk=29358&T=2

[TABLE]
[ROW][C]Regression: S.E.(k) = alpha + beta * Mean(k)[/C][/ROW]
[ROW][C]alpha[/C][C]54.0208961852103[/C][/ROW]
[ROW][C]beta[/C][C]-0.367802970978669[/C][/ROW]
[ROW][C]S.D.[/C][C]0.165739886865321[/C][/ROW]
[ROW][C]T-STAT[/C][C]-2.21915784989973[/C][/ROW]
[ROW][C]p-value[/C][C]0.0448883210996267[/C][/ROW]
[/TABLE]
Source: https://freestatistics.org/blog/index.php?pk=29358&T=2

Globally Unique Identifier (entire table): ba.freestatistics.org/blog/index.php?pk=29358&T=2

As an alternative you can also use a QR Code:

The GUIDs for individual cells are displayed in the table below:

Regression: S.E.(k) = alpha + beta * Mean(k)
alpha	54.0208961852103
beta	-0.367802970978669
S.D.	0.165739886865321
T-STAT	-2.21915784989973
p-value	0.0448883210996267

Regression: ln S.E.(k) = alpha + beta * ln Mean(k)
alpha	20.4643769144706
beta	-3.83278714720105
S.D.	1.71854633310420
T-STAT	-2.23024952738859
p-value	0.0439807736688922
Lambda	4.83278714720105

\begin{tabular}{lllllllll}
\hline
Regression: ln S.E.(k) = alpha + beta * ln Mean(k) \tabularnewline
alpha & 20.4643769144706 \tabularnewline
beta & -3.83278714720105 \tabularnewline
S.D. & 1.71854633310420 \tabularnewline
T-STAT & -2.23024952738859 \tabularnewline
p-value & 0.0439807736688922 \tabularnewline
Lambda & 4.83278714720105 \tabularnewline
\hline
\end{tabular}
%Source: https://freestatistics.org/blog/index.php?pk=29358&T=3

[TABLE]
[ROW][C]Regression: ln S.E.(k) = alpha + beta * ln Mean(k)[/C][/ROW]
[ROW][C]alpha[/C][C]20.4643769144706[/C][/ROW]
[ROW][C]beta[/C][C]-3.83278714720105[/C][/ROW]
[ROW][C]S.D.[/C][C]1.71854633310420[/C][/ROW]
[ROW][C]T-STAT[/C][C]-2.23024952738859[/C][/ROW]
[ROW][C]p-value[/C][C]0.0439807736688922[/C][/ROW]
[ROW][C]Lambda[/C][C]4.83278714720105[/C][/ROW]
[/TABLE]
Source: https://freestatistics.org/blog/index.php?pk=29358&T=3

Globally Unique Identifier (entire table): ba.freestatistics.org/blog/index.php?pk=29358&T=3

As an alternative you can also use a QR Code:

The GUIDs for individual cells are displayed in the table below:

Regression: ln S.E.(k) = alpha + beta * ln Mean(k)
alpha	20.4643769144706
beta	-3.83278714720105
S.D.	1.71854633310420
T-STAT	-2.23024952738859
p-value	0.0439807736688922
Lambda	4.83278714720105

Figure 1

PNG link

Postscript link

PDF link

Figure 2

PNG link

Postscript link

PDF link

Parameters (Session):

Parameters (R input):

par1 = 4 ;

R code (references can be found in the software module):

par1 <- as.numeric(par1)
(n <- length(x))
(np <- floor(n / par1))
arr <- array(NA,dim=c(par1,np))
j <- 0
k <- 1
for (i in 1:(np*par1))
{
j = j + 1
arr[j,k] <- x[i]
if (j == par1) {
j = 0
k=k+1
}
}
arr
arr.mean <- array(NA,dim=np)
arr.sd <- array(NA,dim=np)
arr.range <- array(NA,dim=np)
for (j in 1:np)
{
arr.mean[j] <- mean(arr[,j],na.rm=TRUE)
arr.sd[j] <- sd(arr[,j],na.rm=TRUE)
arr.range[j] <- max(arr[,j],na.rm=TRUE) - min(arr[,j],na.rm=TRUE)
}
arr.mean
arr.sd
arr.range
(lm1 <- lm(arr.sd~arr.mean))
(lnlm1 <- lm(log(arr.sd)~log(arr.mean)))
(lm2 <- lm(arr.range~arr.mean))
bitmap(file='test1.png')
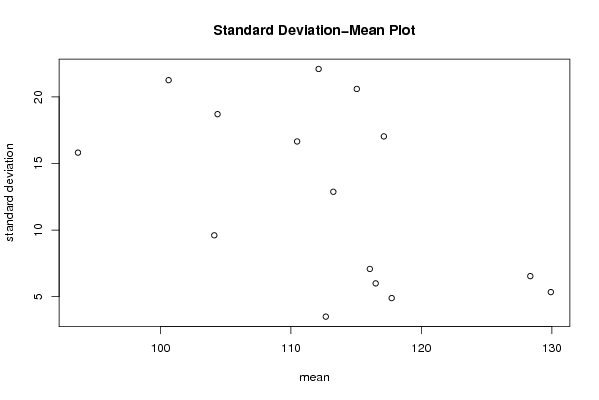
plot(arr.mean,arr.sd,main='Standard Deviation-Mean Plot',xlab='mean',ylab='standard deviation')
dev.off()
bitmap(file='test2.png')
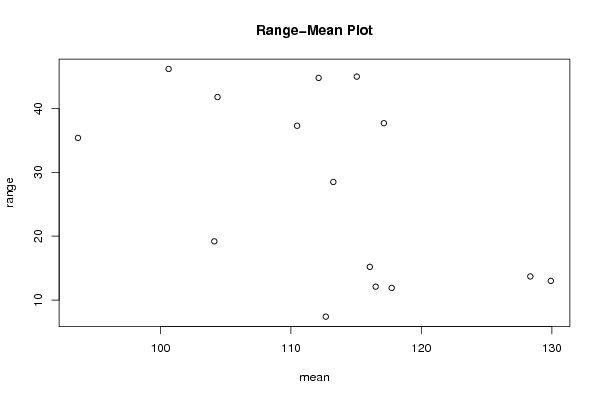
plot(arr.mean,arr.range,main='Range-Mean Plot',xlab='mean',ylab='range')
dev.off()
load(file='createtable')
a<-table.start()
a<-table.row.start(a)
a<-table.element(a,'Standard Deviation-Mean Plot',4,TRUE)
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'Section',header=TRUE)
a<-table.element(a,'Mean',header=TRUE)
a<-table.element(a,'Standard Deviation',header=TRUE)
a<-table.element(a,'Range',header=TRUE)
a<-table.row.end(a)
for (j in 1:np) {
a<-table.row.start(a)
a<-table.element(a,j,header=TRUE)
a<-table.element(a,arr.mean[j])
a<-table.element(a,arr.sd[j] )
a<-table.element(a,arr.range[j] )
a<-table.row.end(a)
}
a<-table.end(a)
table.save(a,file='mytable.tab')
a<-table.start()
a<-table.row.start(a)
a<-table.element(a,'Regression: S.E.(k) = alpha + beta * Mean(k)',2,TRUE)
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'alpha',header=TRUE)
a<-table.element(a,lm1$coefficients[[1]])
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'beta',header=TRUE)
a<-table.element(a,lm1$coefficients[[2]])
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'S.D.',header=TRUE)
a<-table.element(a,summary(lm1)$coefficients[2,2])
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'T-STAT',header=TRUE)
a<-table.element(a,summary(lm1)$coefficients[2,3])
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'p-value',header=TRUE)
a<-table.element(a,summary(lm1)$coefficients[2,4])
a<-table.row.end(a)
a<-table.end(a)
table.save(a,file='mytable1.tab')
a<-table.start()
a<-table.row.start(a)
a<-table.element(a,'Regression: ln S.E.(k) = alpha + beta * ln Mean(k)',2,TRUE)
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'alpha',header=TRUE)
a<-table.element(a,lnlm1$coefficients[[1]])
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'beta',header=TRUE)
a<-table.element(a,lnlm1$coefficients[[2]])
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'S.D.',header=TRUE)
a<-table.element(a,summary(lnlm1)$coefficients[2,2])
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'T-STAT',header=TRUE)
a<-table.element(a,summary(lnlm1)$coefficients[2,3])
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'p-value',header=TRUE)
a<-table.element(a,summary(lnlm1)$coefficients[2,4])
a<-table.row.end(a)
a<-table.row.start(a)
a<-table.element(a,'Lambda',header=TRUE)
a<-table.element(a,1-lnlm1$coefficients[[2]])
a<-table.row.end(a)
a<-table.end(a)
table.save(a,file='mytable2.tab')

Free Statistics

Description of Statistical Computation

Tree of Dependent Computations

Dataset

Tables (Output of Computation)

Figures (Output of Computation)

Input Parameters & R Code